Click on the image below to access an interactive to investigate the formula for the volume of prisms. Follow the onscreen prompts until you reach the summary screen in the interactive.To begin this section, let's take a look back at how to determine the volume of prisms.
Click on the image below to access an interactive to investigate the formula for the volume of prisms. Follow the onscreen prompts until you reach the summary screen in the interactive.
NeedInteractive popup. Assistance may be required.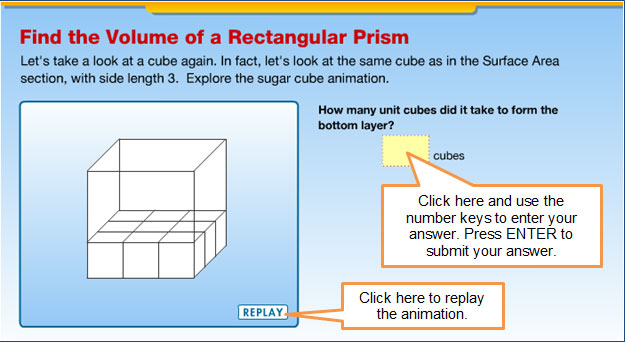 
?
How did you determine the area of the base layer?
Interactive popup. Assistance may be required.One way to determine the area is to multiply the base length by the base width.
How did you determine the total number of cubes it took to fill the large cube?
Interactive popup. Assistance may be required.One way to determine the total number of cubes is to multiply the area of the base by the number of layers (height of the prism).
In the following interactive, drag the appropriate symbols into the appropriate place to build a formula for calculating the volume of any prism.
Now that you’ve written a general formula for the volume of any prism, let’s extend that to write a formula for the volume of a pyramid.
Recall that a prism and a pyramid both have polygonal bases. Let’s consider a prism and a pyramid that have congruent bases and the same height as shown in the image below.
Use the applet below to pour the volume of one pyramid into a prism with a congruent base and the same height. In the applet, select the “Cone -> Tank” option, and click “New Problem” until you have a pyramid and a prism. Use the slider to estimate the height of the liquid inside the prism once it is poured from the pyramid into the prism. Then, click “Pour” to pour the liquid and check your estimate. Repeat this for three or four different pyramids until you see a pattern.
NeedInteractive popup. Assistance may be required.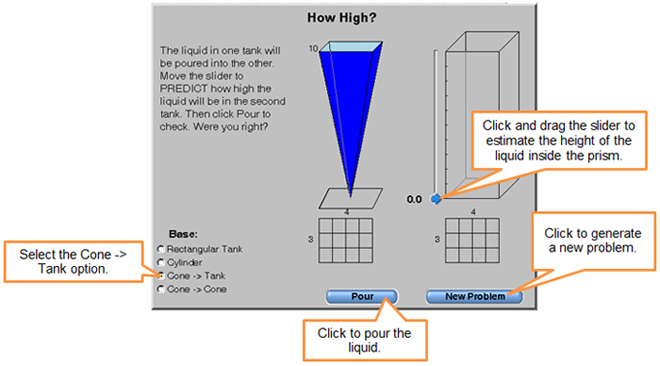 
?
If the height of the prism is 10 units, about how high will the liquid from the pyramid fill the prism?
Interactive popup. Assistance may be required.About 3.3 units
In general, for any height of corresponding pyramids and prisms, about how high will the liquid from the pyramid fill the prism?
Interactive popup. Assistance may be required.About 1 over 3 1 3 of the height of the prism
In the following interactive, drag the appropriate symbols into the appropriate place to build a formula for calculating the volume of any pyramid.
Let’s suppose a prism and pyramid have congruent bases and congruent heights. If you were to fill the pyramid with water and empty it into the prism, how many pyramids would it take to completely fill the prism?
How is the volume formula for a pyramid related to the volume formula for a prism?
Match each figure below with an expression that could be used to determine its volume. Move the expression to see the original formula from which it comes.