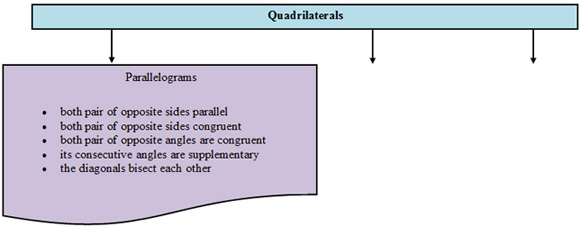
In this section, you are going to continue investigating quadrilaterals that have unique characteristics.

A parallelogram is a quadrilateral that has:

• both pair of opposite sides parallel
• both pair of opposite sides congruent
• both pair of opposite angles are congruent
• its consecutive angles are supplementary
• the diagonals bisect each other

Now that you understand parallelograms, you can start working on the flow chart for quadrilaterals. You can fill in what you know so far about parallelograms.

Look at the parallelogram below.

Let’s think about what properties would need to be present to know that this parallelogram is also a rectangle.

By definition, a rectangle is a parallelogram with four right angles. With this in mind, let’s see what our rectangle would look like:

• Click to start the animation and watch as the vertex at ∠A changes from 120° to 90°.
• Notice that once you reach 90° for the measure of ∠A, the right angle symbol appears at the vertex of ∠D.
• Now you are showing that you have a parallelogram with four right angles so you have a rectangle.

You could say that a rectangle is always a parallelogram, but a parallelogram is not always a rectangle.

Using this applet, http://www.mathopenref.com/rectanglediagonals.html:

1. To the right on the applet click the box next to both, both diagonals should appear.
2. Select each vertex one at a time to change the lengths of the sides of the rectangle.

In your notes, answer the following questions from the Investigate section:

1. Compare the lengths of diagonals AC and BD. What do you notice?
2. Recall that a rectangle is also a parallelogram, so its diagonals also have the properties of a parallelogram's diagonals. So, what else can you say about the diagonals of this rectangle?
3. Formulate the Rectangle Diagonals Conjecture: The diagonals of a rectangle are _____, and _____.
4. A square is a parallelogram, as well as both a rectangle and a rhombus. Use what you know about the properties of these three quadrilaterals to formulate the Square Diagonals Conjecture: The diagonals of a square are _____, _____, and _____.

Knowing that a rectangle is a parallelogram, what are two important properties of the diagonals of a rectangle? Use a picture to illustrate your response.

Interactive popup. Assistance may be required.

Possible Response

For rectangles, the diagonals bisect each other as they do for all parallelograms. The diagonals of a rectangle are also congruent.

So, you know that a rectangle is always a parallelogram, but a parallelogram is not always a rectangle. Not bad! Rectangles are easy! Let’s add rectangles to our quadrilateral flow chart:

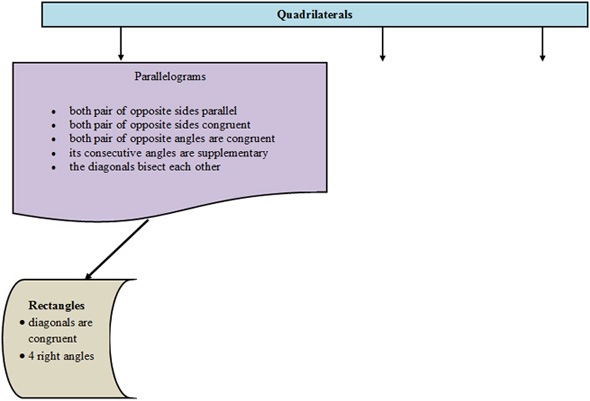
An important property of a rectangle that is different from other parallelograms is that the diagonals of a rectangle are congruent.

This means that if you have a parallelogram with congruent diagonals then you know the parallelogram is a rectangle.

If you are given ABCD and you are given that AC = 24 and BD = 3(2x-5), what value of x would be necessary in order to prove that ABCD is a rectangle?

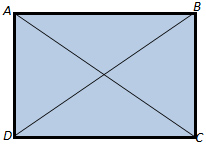
First things first! If ABCD is a rectangle, then AC ≅ BD. That means that AC = BD.

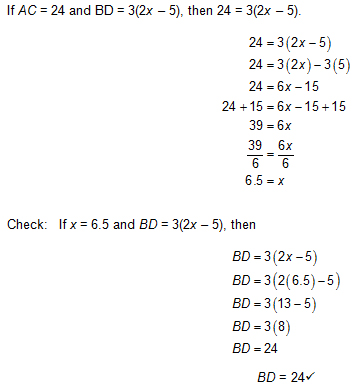
Conclusion: If x = 6.5, then BD = 24 and AC ≅ BD. This means that ABCD is a rectangle since the diagonals are congruent.