One way that you can solve one-variable inequalities is to use a graph or a table.

Consider the situation shown below.

• Write an inequality that could be used to determine possible values of x for this situation.

  Interactive popup. Assistance may be required.

  Need a hint?

  Linear functions have a constant term, which can be a fixed fee, and a rate of change, or slope. In this situation, what is the fixed fee, and what is the rate charged per ticket?

  Interactive popup. Assistance may be required.

  Check Your Answer

  35.50x + 12 ≤ 438

• Use a graphing calculator, online graphing calculator, or a graphing calculator app to generate a table of values for the equation Y1 = 35.50x + 12 and the equation Y2 = 438. Copy the table below into your notes and use the information from your graphing calculator to complete the table.
  

  x	Y1= 35.50x + 12	Y2 = 438
  8
  9
  10
  11
  12
  13
  14

You can use an online graphing calculator such as meta-calculator.

Interactive popup. Assistance may be required.

Click
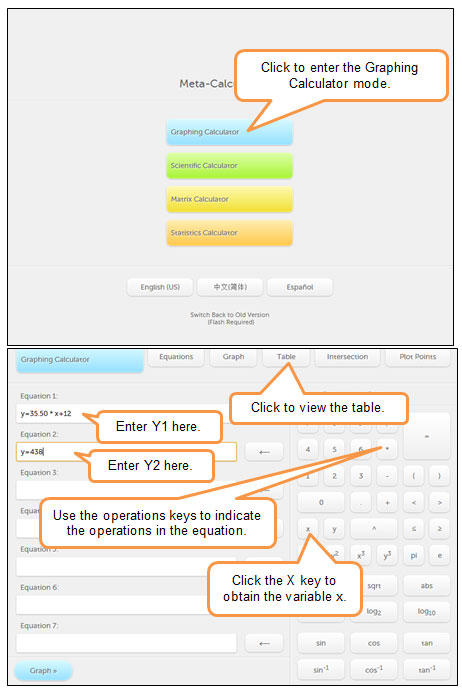
to see instructions on how to use this online calculator to generate a table of values.

Interactive popup. Assistance may be required.

Check Your Answer

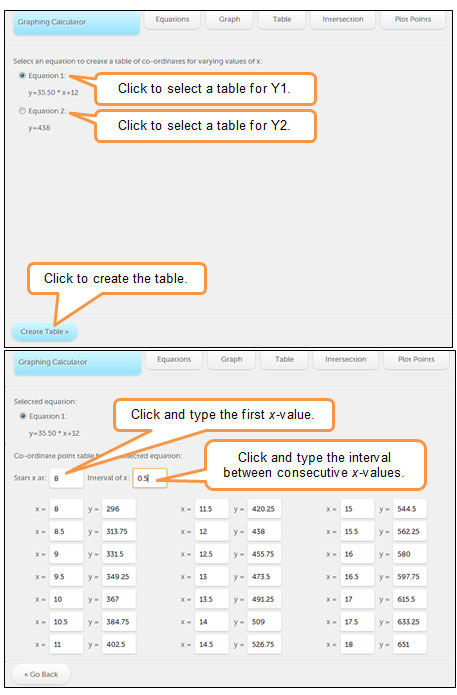
x	Y1 = 35.50x + 12	Y2 = 438
8	296	438
9	331.5	438
10	367	438
11	402.5	438
12	438	438
13	473.5	438
14	509	438


• In the table, what value of x generates the same value for both Y1 and Y2? (This value of x makes the equation 35.50x + 12 = 438 true.)

  Interactive popup. Assistance may be required.

  Need a hint?

  Read down the Y1 and Y2 columns until you see the same number side by side.
  Interactive popup. Assistance may be required.

  Check Your Answer

  x = 12

• For values of x less than where Y1 = Y2, how do the values of Y1 and Y2 compare?

  Interactive popup. Assistance may be required.

  Need a hint?

  Read the chart upward from x = 12. Would you say that Y1 is less than Y2 or greater than Y2?
  Interactive popup. Assistance may be required.

  Check Your Answer

  Y1 < Y2

• For values of x greater than where Y1 = Y2, how do the values of Y1 and Y2 compare?

  Interactive popup. Assistance may be required.

  Need a hint?

  Read the chart downward from x = 12. Would you say that Y1 is less than Y2 or greater than Y2?
  Interactive popup. Assistance may be required.

  Check Your Answer

  Y1 > Y2

• Which set of x-values seem to satisfy the inequality 35.50x + 12 ≤ 438?

  Interactive popup. Assistance may be required.

  Need a hint?

  Read the chart to identify the x-values where Y1 = Y2 and Y1 < Y2?
  Interactive popup. Assistance may be required.

  Check Your Answer

  x ≤ 12

Pause and Reflect

How would the possible values for x change if the budget for the Spanish Club had been $367 instead of $438?

Interactive popup. Assistance may be required.

Check Your Answer

The Spanish Club could have spent no more than $367, so change Y2 to 367 and look for the x-values where Y1 ≤ Y2. We can see that x ≤ 10.

How would your solution strategy be different if the Spanish Club decided to spend at least $400 on concert tickets?

Interactive popup. Assistance may be required.

Check Your Answer

If the situation were "at least $400," then the inequality would be greater than or equal to $400. Instead of looking for x-values where Y1 ≤ Y2, look for x-values where Y1 ≥ Y2.

---

Practice

1. Juliette used a table of values to solve the inequality 4(x – 10) > 8. She entered Y1 = 4(x – 10) and Y2 = 8 into her graphing calculator to generate the table below.
   

   x	Y1	Y2
   9	-4	8
   10	0	8
   11	4	8
   12	8	8
   13	12	8
   14	16	8
   15	20	8

   Based on the table, what would the solution be to the inequality, 4(x – 10) > 8? Interactive popup. Assistance may be required.

   Need a hint?

   Determine the x-values where Y1 > Y2, but Y1 ≠ Y2.

   Interactive popup. Assistance may be required.

   Check Your Answer

   x > 12

2. Antoine used a table of values to solve the inequality 4.3(2x + 1.4) < 49.02. He placed Y1 = 4.3(2x + 1.4) into his graphing calculator and obtained the table of values shown below.

   x	Y1
   3	31.82
   4	40.42
   5	49.02
   6	57.62
   7	66.22
   8	74.82

   Based on the table, what would be the solution to the inequality 4.3(2x + 1.4) < 49.02?

   Interactive popup. Assistance may be required.

   Need a hint?

   Determine the x-values where Y1 < 49.02, but Y1 ≠ 49.02.
   Interactive popup. Assistance may be required.

   Check Your Answer

   x < 5

3. Celsa used a table of values to solve the inequality 2x + 3.5 ≥ 3(x – 17). She entered Y1 = 2x + 3.5 and Y2 = 3(x – 17) into her graphing calculator to generate the table below.

   x	Y1	Y2
   53	109.5	108
   53.5	110.5	109.5
   54	111.5	111
   54.5	112.5	112.5
   55	113.5	114
   55.5	114.5	115.5
   56	115.5	117

   Based on the table, what would the solution be to the inequality 2x + 3.5 ≥ 3(x – 17)?

   Interactive popup. Assistance may be required.

   Need a hint?

   Determine the x-values where Y1 ≥ Y2.
   Interactive popup. Assistance may be required.

   Check Your Answer

   x ≥ 54.5