In the last section, you used a balance scale model to set up and solve two-step equations. In this section, you will use a different model composed of cups and counters.

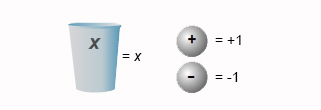
For the cups and counters model, a cup represents the variable, usually x, and counters are used to represent numbers.

key for cups and counters model

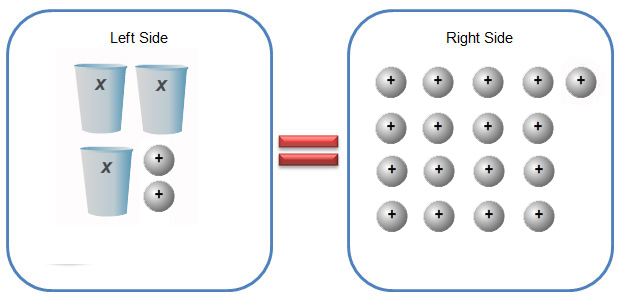
Consider the equation 3x + 4 = 13. This equation can be modeled with cups and counters with 3 cups and 4 positive unit counters on the left side of an equal sign and 13 positive counters on the right side of an equal sign.

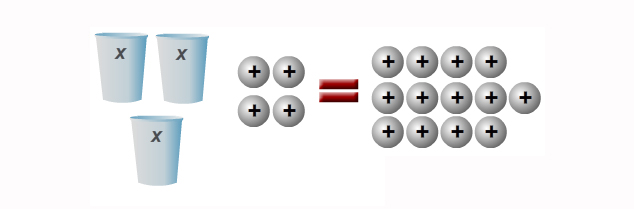
cups and counters model for 3x + 4 = 13

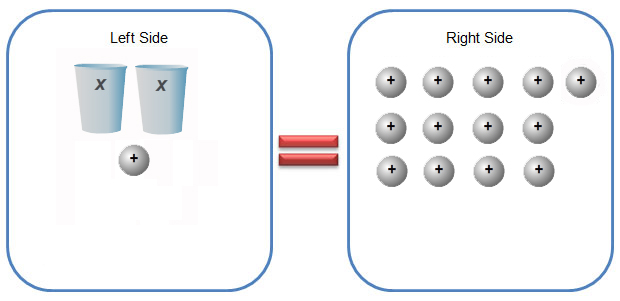
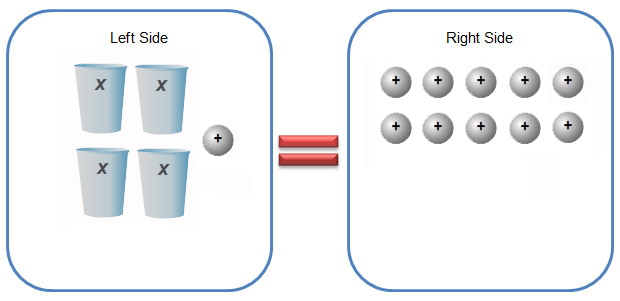
Interactive exercise. Assistance may be required. Click through the interactive below to see how Sandy used cups and counters to solve this equation. Click the Next arrow to step through how Sandy did it.

Interactive exercise. Assistance may be required. Click and drag to add cups and/or counters to the model. Use the cups and counters model below to set up and solve each of the equations shown.

Pause and Reflect

When you are dividing counters evenly among the cups, what do you do if there is a counter left over?

Interactive popup. Assistance may be required.

Check Your Answer

Left over counters can be broken into fractional pieces. If you are dividing by 2, break them into 2 pieces, and distribute the pieces evenly. If you are dividing by 3, break them into 3 pieces, and distribute the pieces evenly.Close Pop Up

If you have negative counters instead of positive counters, what action do you need to take instead of removing counters from both sides of the model?

Interactive popup. Assistance may be required.

Check Your Answer

To remove counters with opposite signs, you need to add zero pairs to cancel the negative counters.Close Pop Up

Practice

For questions 1 – 3, use the cups and counters model to determine the value of x.

  1. 3x + 2 = 17
    Interactive popup. Assistance may be required.

    Need a hint?

    How many unit counters do you need to remove from both sides of the model in order to get the 3 cups by themselves?Close Pop Up
    Interactive popup. Assistance may be required.

    Check Your Answer

    x = 5Close Pop Up
  2. 2x + 1 = 13
    Interactive popup. Assistance may be required.

    Need a hint?

    How many unit counters do you need to remove from both sides of the model in order to get the 2 cups by themselves?Close Pop Up
    Interactive popup. Assistance may be required.

    Check Your Answer

    x = 6Close Pop Up
  3. 4x + 1 = 10
    Interactive popup. Assistance may be required.

    Need a hint?

    How many unit counters do you need to remove from both sides of the model in order to get the 4 cups by themselves? If you have counters left over, into what fractional parts can you break them in order to distribute the fractional parts evenly among the 4 cups?Close Pop Up
    Interactive popup. Assistance may be required.

    Check Your Answer

    x = 21 over 2 1 4 Close Pop Up