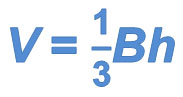
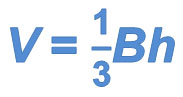
The volume formula for any pyramid relates the volume, V, to the area of the base, B, and the height of the prism, h.
The way that you might choose to approach a volume problem involving a pyramid depends on the shape of the base. In this section, you will focus on rectangular pyramids, or pyramids with rectangular bases.

Source: Flickr - Gaspa - Giza, la piramide grande, Francesco Gasparetti, Wikimedia Commons
The Cheops Pyramid in Egypt is a square pyramid, or a pyramid whose base is a square. Ancient Egyptians constructed the Cheops Pyramid.

Source: Kukulcan, Chichén Itzá, Kyle Simourd, Wikimedia Commons
The Kukulcan Pyramid at Chichen Itza, Mexico, is also an example of a square pyramid. Ancient Mayans constructed the Kukulcan Pyramid.
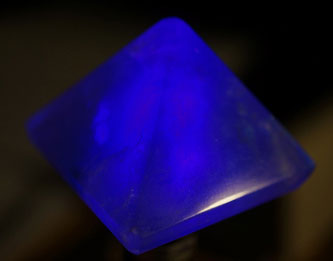
Source: Fluorite pyramid and blue laser, Mike Lewinski, Flickr Commons
A pyramid of fluorite, a mineral found throughout the world, can be used to refract the light from a blue laser and create special visual effects!
Rectangular pyramids appear frequently in art and architecture. Because they are so common, it is important to be able to calculate the volume of a rectangular pyramid.
A problem-solving process may help you when you encounter problems related to a rectangular pyramid.
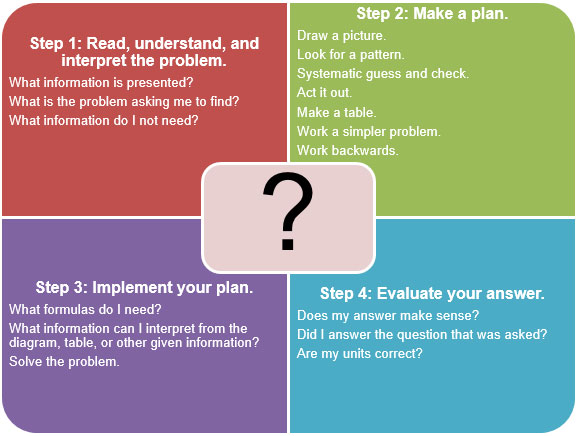
Consider the problem shown below.
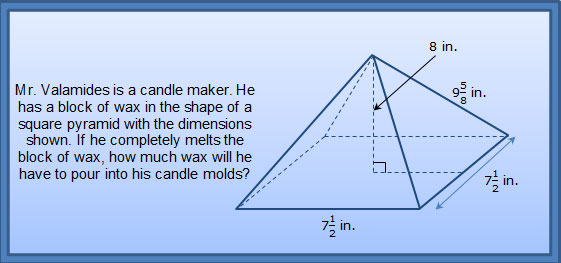
![]() Click on the Begin button to begin the interactive below, and see how Sabrina used a problem-solving model to solve this problem.
Click on the Begin button to begin the interactive below, and see how Sabrina used a problem-solving model to solve this problem.
If you were solving the same problem as Sabrina, what solution strategy would you have selected?

Sabrina used rounding to check her answer for reasonableness. What is another way to know that your answer is reasonable?


Source: A Hindu temple at Chhattarpur temple complex, Delhi, Alicia Nijdam, Wikimedia Commons
Interactive popup. Assistance may be required.
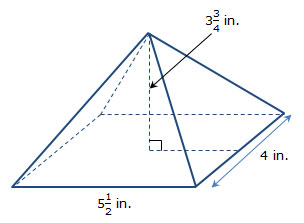
Use the formula, V = 1 over 3 1 3 Bh.
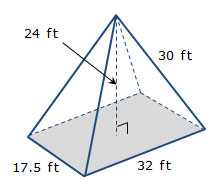
What is the volume of the interior of the attic space?