In the introduction, you reviewed area formulas that you can use to calculate the area of particular polygons and circles. In the real world, not every figure can be cleanly classified as a rectangle, parallelogram, triangle, or trapezoid. Consider the following real-world objects for which it is useful to be able to determine the area.

Source: Landscape Design Advisor, Flickr

Source: Liben(121), Dezidor, Wikimedia Commons

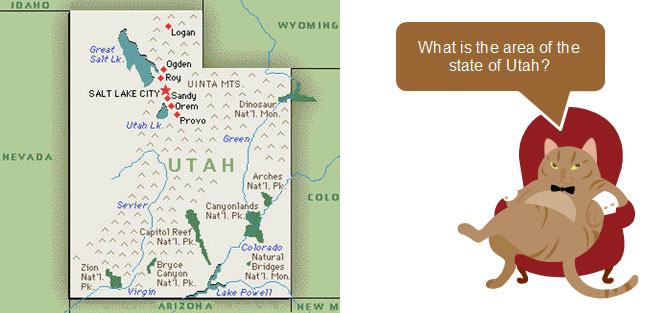
In order to calculate the area of these figures and many more, you need to be able to solve problems that involve composite figures.

The border of a playground is shown below. Click the Animate button to see how one student partitioned the figure.

One way that you can break down a figure into triangles, squares, and parallelograms is to use tangrams. Tangrams are tiles from a Chinese puzzle that can be rearranged to create geometric designs.

Use the tangrams pieces in the interactive below to recreate the composite figures beneath the link to the tangrams interactive. Click on the image to open the interactive, which will open in a new browser tab or window. Then, click and drag on the tangram pieces until you have recreated the composite figure. There may be more than one possible answer.

Interactive popup. Assistance may be required.

Need additional directions?

Interactive popup. Assistance may be required. Check Your Answer

Interactive popup. Assistance may be required. Check Your Answer

Interactive popup. Assistance may be required.

Check Your Answer

Pause and Reflect

When you are partitioning a composite figure into triangles, what patterns should you look for?

Interactive popup. Assistance may be required.

Check Your Answer

Look for perpendicular lines that are connected with a diagonal side.

If you were partitioning a figure into rectangles, what would you need to look for?

Interactive popup. Assistance may be required.

Check Your Answer

Rectangles are figures with both pair of congruent opposite sides and four right angles, so look for pairs of opposite sides that could be congruent and perpendicular lines.

Practice

For questions 1 – 3, partition the composite figure into a combination of rectangles, triangles, parallelograms, trapezoids, or circles (including semicircles or quarter circles). There may be more than one possible answer. You may assume that angles appearing to be right angles are right angles.

1. 
   
   
   Interactive popup. Assistance may be required.

   Need a hint?

   Rectangles have four right angles and opposite sides that are congruent. Look for ways to partition the figure around right angles.
   Interactive popup. Assistance may be required.

   Check Your Answer

   Possible solution:
   
2. 
   
   
   Interactive popup. Assistance may be required.

   Need a hint?

   Where would you locate the diameter of a semicircle?
   Interactive popup. Assistance may be required.

   Check Your Answer

   Possible solution:
   
3. 
   
   
   Interactive popup. Assistance may be required.

   Need a hint?

   How could you draw vertical lines to create right angles inside the figure?
   Interactive popup. Assistance may be required.

   Check Your Answer

   Possible solution: