In this resource, we will look more closely at one method for solving problems that involve proportional relationships — multiplication by a constant.

Read the following problem and answer the questions below. Record your answers on a separate piece of paper or using your notes.

A man is planning to grow chili peppers in his garden. He knows that each plant will produce eight chili peppers. He wants to know how many peppers he will grow based on the number of plants.

Copy the following table into your notes and fill in the missing information.

Click here to see the completed table.

Plants	Process	Peppers
1	1(8)	8
2	2(8)	16
3	3(8)	24
4	4(8)	32
5	5(8)	40
8	8(8)	64
10	10(8)	80

What do all the expressions in the 'Process' column have in common?

Interactive popup. Assistance may be required. Check Your Answer They all show the number of plants multiplied by 8.

Write a rule for determining the number of peppers, if you know the number of plants.

Interactive popup. Assistance may be required. Check Your Answer To solve for the number of peppers, multiply the number of plants by 8.

Now write your rule as an equation. Since both "peppers" and "plants" start with the letter p, you will have to use different letters for your variables. In your equation, use y for the number of peppers and x for the number of plants.

Interactive popup. Assistance may be required. Check Your Answer y = 8x

In this problem we solved a proportional relationship by multiplying by a constant:

Instead of writing out the word "constant," mathematicians use the letter k.

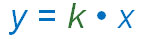
What was the constant in the pepper plant example?

Interactive popup. Assistance may be required. Check Your Answer 8

The constant that you use does not have to be a whole number. It can be any number including fractions, decimals, percents, or irrational numbers like √2.

On track and field day at an elementary school, the teacher wants to give her class a treat, so she buys popsicles. The popsicles cost $0.45 each. Fill in the following table to determine the equation for the cost c, of buying p, popsicles.

Click here to see the completed table.

Popsicles	Process	Cost
1	1(0.45)	$0.45
2	2(0.45)	$0.90
3	3(0.45)	$1.35
5	5(0.45)	$2.25
10	10(0.45)	$4.50
p	p(0.45)	$0.45p

What is the rule for filling in the table?

Interactive popup. Assistance may be required. Check Your Answer Multiply the number of popsicles by 0.45 to find the cost.

What is the constant in this problem?

Interactive popup. Assistance may be required. Check Your Answer 0.45

Write an equation that could be used to solve this problem.

Interactive popup. Assistance may be required. Check Your Answer c = 0.45p

The teacher has 22 students, how much will she pay for the popsicles?

Interactive popup. Assistance may be required. Check Your Answer c = 0.45(22)
c = 9.9
She will pay $9.90 for the popsicles.

Both of these problems could be modeled using proportional relationships because when one variable changed, the other changes by the same factor. We solved them by finding a constant of proportionality between the two variables and set up the equation y = constant • x. The equation may also be written in the form, y = kx where k represents the constant of proportionality.