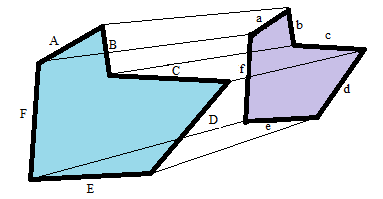
One common situation that can be represented with proportional relationships is analyzing similar figures. Similar figures are different sizes of the exact same shape.
When setting up a proportion for a pair of similar figures, you can only compare the lengths of corresponding sides. Corresponding sides are the matching sides of the similar figures. In the picture above, the corresponding sides are labeled with upper and lower case of the same letter.
![]()
Select the matching pairs by clicking on the tile that represents one of the corresponding sides or one of the equivalent ratios:
One example of using similar figures is making scale drawings or models.
Read the following, and answer the questions below.

An architect is drawing a scale picture of a house. The dimensions of the actual rooms and the rooms in the picture are listed in the table below.
Copy the table into your notes, and fill in the missing information.
| Actual Measurement | Process | Picture Measurement | ||
|---|---|---|---|---|
| Living Room | Length | 20 Feet | 20 ( 1 over 4 1 4 ) = 5 | 5 inches |
| Width | 12 Feet | 12 ( 1 over 4 1 4 ) = 3 | 3 inches | |
| Dining Room | Length | 18 Feet | 4.5 inches | |
| Width | 10 Feet | |||
| Kitchen | Length | 12 Feet | ||
| Width | 16 Feet | |||
| Bedroom | Length | 12 Feet | ||
| Width | 10 Feet | |||
| Actual Measurement | Process | Picture Measurement | ||
|---|---|---|---|---|
| Living Room | Length | 20 Feet | 20 ( 1 over 4 1 4 ) = 5 | 5 inches |
| Width | 12 Feet | 12 ( 1 over 4 1 4 ) = 3 | 3 inches | |
| Dining Room | Length | 18 Feet | 18 ( 1 over 4 1 4 ) = 4.5 | 4.5 inches |
| Width | 10 Feet | 10 ( 1 over 4 1 4 ) = 2.5 | 2.5 inches | |
| Kitchen | Length | 12 Feet | 12 ( 1 over 4 1 4 ) = 3 | 3 inches |
| Width | 16 Feet | 16 ( 1 over 4 1 4 ) = 4 | 4 inches | |
| Bedroom | Length | 12 Feet | 12 ( 1 over 4 1 4 ) = 3 | 3 inches |
| Width | 10 Feet | 10 ( 1 over 4 1 4 ) = 2.5 | 2.5 inches | |

What do you notice is constant in the table?
Interactive popup. Assistance may be required. The factor that is used in the process column is constant.
What proportional relationship could you use to relate p, a measurement in the picture, with a, an actual measurement?
Interactive popup. Assistance may be required. 1 over 4 1 4 = p over a p a
Given that y → picture measurement, k → 1 over 4 1 4 and x → actual measurement use the variables ‘p’ and ‘a’ to rewrite the proportional relationship as an equation that looks like y = kx.
Interactive popup. Assistance may be required. p = 1 over 4 1 4 a
If the architect were to measure one of the bathrooms in the house to be 10 feet long by 8 feet wide, what would the dimensions of the bathroom be in the picture?
Interactive popup. Assistance may be required. The picture would be 2.5 inches long by 2 inches wide.
If the architect were to measure the length of a second bedroom in the house to be 15 feet long, what would the width of the second bedroom be in the picture?
Interactive popup. Assistance may be required. This cannot be calculated because the width of the actual room is not stated.