WHEW! That is a lot of information about parallelograms! Let's look back at all you have learned.
If a quadrilateral is a parallelogram:
Sometimes you have to show that there is enough information to prove that a quadrilateral is a parallelogram. Considering all that you have learned so far, let's look at the conditions necessary to show that a quadrilateral is a parallelogram:
Condition 1
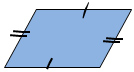
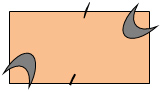
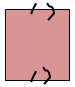
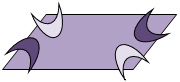
If one pair of opposite sides of a quadrilateral are parallel and congruent, then the quadrilateral is a parallelogram.
Condition 2
If both pair of opposite sides of a quadrilateral are congruent, then the quadrilateral is a parallelogram.
Condition 3
If both pair of opposite angles of a quadrilateral are congruent, then the quadrilateral is a parallelogram.
Condition 4
If the diagonals of a quadrilateral bisect each other, then the quadrilateral is a parallelogram.
![]() Let's practice recognizing these four conditions.
Let's practice recognizing these four conditions.
Look at each diagram below and tell whether or not it is a parallelogram. Be sure to support your answer with one of the conditions above. Click on "Answer" to see if you are correct.
Is it a parallelogram? |
|
 |
|
 |
|
 |
|
 |
|
 |
|
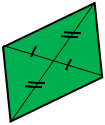 |