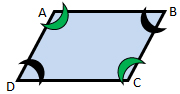
Look at the parallelogram to the right.
∠A and ∠C are opposite angles, therefore ∠A≅∠C
∠B and ∠D are opposite angles, therefore∠B≅∠D
In the previous section, you investigated four important properties of parallelograms:
Theorem 1: If a quadrilateral is a parallelogram, then its opposite sides are congruent.
Theorem 2: If a quadrilateral is a parallelogram, then its opposite angles are congruent.
Theorem 3: If a quadrilateral is a parallelogram, then its consecutive angles are supplementary.
Theorem 4: If a quadrilateral is a parallelogram, then its diagonals bisect each other.
In this section, you will apply those theorems and properties to solve problems.
Theorem 2: If a quadrilateral is a parallelogram, then its opposite angles are congruent.
Look at the parallelogram to the right.
∠A and ∠C are opposite angles, therefore ∠A≅∠C
∠B and ∠D are opposite angles, therefore∠B≅∠D

If we know that the m∠D = 3x + 5 and m∠B = 4x - 15, we can find the measure of each angle. Since the angles are congruent, we know they have the same measure. We can set the two expressions equal and solve for x. Once we know the value of x, you can substitute the value of x into each expression to verify that the two angles have the same measure and are congruent.
4x - 15 = 3x + 5 |
|
4x - 15 + 15 |
3x+ 5 + 15 |
4x |
3x + 20 |
4x - 3x |
3x - 3x + 20 |
x |
20 |
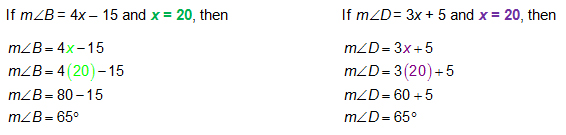
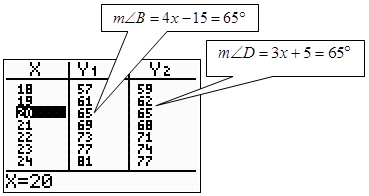
TIP: Check your answer using a graphing calculator:
You Try One!

![]() Scroll your mouse pointer over each blank below to check your work:
Scroll your mouse pointer over each blank below to check your work:
Now you have the value of x, substitute this value into the expression that represents m∠K.
Interactive roll-over image. Assistance may be required.
Theorem 3: If a quadrilateral is a parallelogram, then its consecutive angles are supplementary.
If you know that m∠A = 3x + 15and m∠B = 2x + 5, you can find the measure of each angle of the parallelogram.
m∠A + m∠B = 180o
Step 1 3x + 15 + 2x + 5 = 180
Step 2 5x + 20 = 180
Step 3 5x + 20 - 20 = 180 - 20
Step 4 5x = 160
Step 5
numerator 5x denominator 5
5x
5
numerator 160 denominator 5
160
5
Step 6 x = 32
You've Found the Value of x But You're Not Done Yet!
If m∠A = 3x + 15and x = 32, then:
m∠A = 3x + 15
m∠A = 3(32) + 15
m∠A = 96 + 15
m∠A = 111o
If m∠B = 2x + 5 and x = 32, then:
m∠B = 2x + 5
m∠B = 2(32) + 5
m∠B = 64 + 5
m∠B = 69o
You found the angle measures, but are they really supplementary? Let's check and be sure.
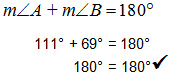
Theorem 4: If a quadrilateral is a parallelogram, then its diagonals bisect each other.
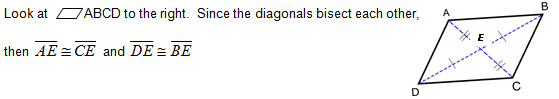
Source: Parallelogram, Wikimedia
If we know that AE = 3x - 5 and CE = x + 25, finding the length AC is a breeze.
Since the diagonals of a parallelogram bisect each other, we know that AE = CE. This of course means that 3x - 5 = x + 25. Let's solve for x and then find AC.

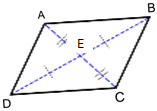
ABCD is a quadrilateral. If AE = 2x - 1 and CE = x + 7, find the value of x so that ABCD is a parallelogram, then find AC.
Show all of your work in your notes.