Finding the total surface area of a composite figure is similar to finding the surface area of 3-dimensional figures such as prisms, cylinders, pyramids, cones, and spheres. These shapes are combined to make 3-dimensional figures called composites figures .

Video segment. Assistance may be required.

Source: Surface Area of Composite Figures (Prisms), TenMarksInstructor, You Tube

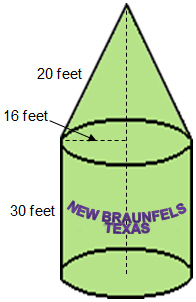
Radius =18 ft, Ht cylinder =30 ft, Ht cone =20 ft

You Try It!

The figure at right represents the water tower for a town in Texas. It is a composite of a cylinder and a cone . The city council has decided to repaint the entire outer surface of the water tower.

Determine the surface area that will be painted.

Identify the Interactive popup. Assistance may be required. hidden surfaces... The base of the cone and the top base of the cylinder Close Pop Up

Lateral Area of cone... LA = πrl
≈ 3.14(16 feet)(20 feet)
≈ 1005 ft2 Close Pop Up

Lateral Area of cylinder... LA = 2πrh
≈ (2)3.14(16 feet)(30 feet)
≈ 3,016 ft2 Close Pop Up

Area of Base of cylinder... A = πr2
≈ 3.14(16 feet)2
≈ 804 ft2 Close Pop Up

Total surface area... SA = LAcone + LAcylinder + Abase
≈ 1005 ft2 + 3016 ft2 + 804 ft2
≈ 4825 ft2 Close Pop Up

Anytime the surface area of a composite figure is found, ask if the top or bottom is open or the connection of the two shapes. If there is an opening or a common side, only the areas of the enclosed sides or non-common sides are found.