m∠AOB = m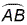
m∠APB
In this part of the lesson, you will investigate the relationship between the measure of an inscribed angle and its corresponding central angle.
Explore the relationship between the measure of an inscribed angle and its corresponding central angle. First, create a table similar to the one below in your notes.
m∠AOB = m |
m∠APB |

![]() Using the interactive applet, http://www.mathopenref.com/arccentralangletheorem.html, move points A, B and P around the circle one at a time, compare the two angle measures and write down at least 4 entries in the table.
Using the interactive applet, http://www.mathopenref.com/arccentralangletheorem.html, move points A, B and P around the circle one at a time, compare the two angle measures and write down at least 4 entries in the table.
Journal Entry:
Both angle measures were provided, compare the angle measures to the arc measure. Would the angle measures and arc measures change if the radius of the circle changed?
