Now, let's investigate how the rule for perimeter changes when we consider other regular polygons, including squares, hexagons, and more.
![]() In the Perimeter of a Polygon Interactive, you can also change the number of sides of the figure by clicking on "more" to add more sides to the regular polygon, or "less" to reduce the number of sides in the regular polygon as highlighted in the picture below.
In the Perimeter of a Polygon Interactive, you can also change the number of sides of the figure by clicking on "more" to add more sides to the regular polygon, or "less" to reduce the number of sides in the regular polygon as highlighted in the picture below.
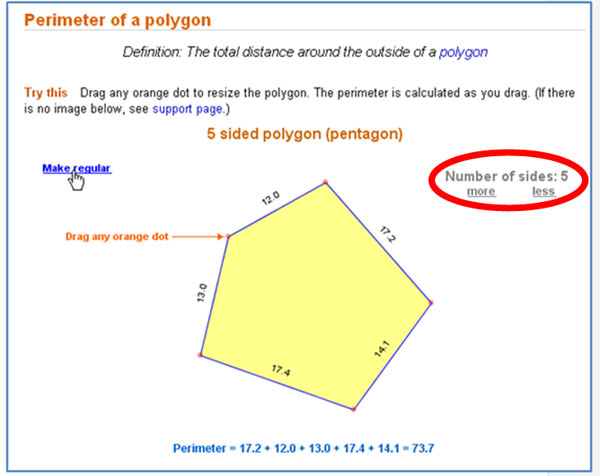
Source: Perimeter of a Polygon, Math Open Reference
Generate values for the side length and perimeter of several different regular polygons. Include at least one pentagon, one hexagon, one quadrilateral, and one polygon with a different number of sides. The first line is filled in for you as an example. Record your results in a table like the one shown. You may create the table in your notes.
| Number of Sides | Side Length | Process to Find Perimeter | Perimeter |
| 5 | 14.1 | 14.1 + 14.1 + 14.1 + 14.1 + 14.1 = 5(14.1) | 70.5 |
| n | s | P=___________ |
Let n represent the number of sides of a regular polygon, s represent the length of one side of the regular polygon, and P represent the perimeter of the regular polygon.
In your notes, answer the following questions: How did you use the table and graph to generate the algebraic expression for this geometric property of polygons? What other features of a graphing calculator could you use to generate algebraic expressions for this geometric property?