Mathematics is a continuously changing and evolving field of study, even today.  In the late 1800s and early 1900s a Russian mathematician named Herman Minkowski proposed a type of plane geometry that later came to be referred to as "taxicab geometry."  In this new geometry, points were located on a Cartesian coordinate plane, but only at intersections of two grid lines.  Minkowski measured the distance between points along the grid lines in the Cartesian plane, rather than diagonally across the grid’s squares.  The shortest distance between two points on the grid, then, was not a straight line, but a series of connected line segments … sort of stair steps. This geometry became known as "taxicab geometry" because the geometer was allowed to travel only along grid lines parallel to the x and y axes, like traveling in a taxicab along the streets of a large city; no cutting through buildings in a cab!

Click on the image to the right to go to the taxicab geometry applet, where you will go in search of a treasure.  The treasure is at the intersection of two streets.  You choose your starting location or intersection by selecting the names of the cross-streets from the drop-down menus above the map.  You can then move from your starting location to any other intersection on the map, again by selecting the names of the cross-streets from the drop-down menus above the map.   The objective of the game is to find the treasure in the fewest steps and to travel the fewest extra “blocks” to get to the treasure.  For example, after picking your starting point, you find that your treasure is 5 blocks away and if you travel 8 blocks before finding the treasure, then you traveled 3 blocks too many.

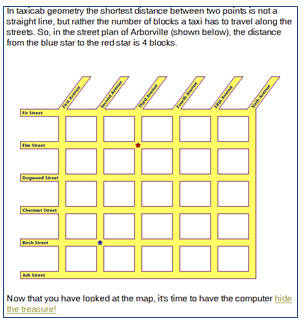If your opponent’s treasure is 3 blocks from his or her starting point, but he or she travels 8 blocks to find the treasure, that is 5 blocks too many.  

Even though your opponent’s treasure was closer to his or her starting point, the opponent had a harder time finding the treasure.  Therefore, you win because you went fewer blocks out of the way to find the treasure.  Way to go!

Now, go to the bottom of the map and click “hide the treasure.”  Select your starting intersection and the treasure!  Good luck!