When we think of geometry, we think of a course in high school that is about things like parallel and perpendicular lines, polygons and circles, axioms, postulates, theorems, and proofs.  Geometry includes a lot of new vocabulary words and was “invented” by a Greek mathematician named Euclid a long time ago. 

There are actually many geometries.  The geometry of Euclid, or Euclidean geometry, is a type of “plane” geometry because all of the rules that govern this geometry occur for figures in a plane, or flat surface.  We know that not all surfaces are flat, however.  Some are curved – in many different ways.  That really complicates what we know about the plane geometry of Euclid.  These geometries have their own set of rules, or axioms, postulates, and theorems. 

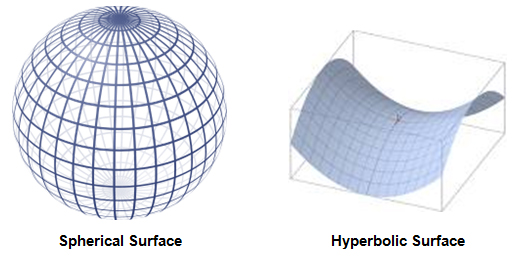
About 300 BC, almost as soon as Euclid had completed his thirteen books, called The Elements, which outlined the axioms, postulates, and theorems of his plane geometry, other mathematicians began to question and speculate.  They wondered whether or not these rules were valid for other surfaces such as spherical and hyperbolic surfaces shown below. 

It was not until over 2000 years later – the 1800s – that other geometries such as spherical and hyperbolic became generally accepted in the mathematics community.  You can read more about non-Euclidean geometries here.