Untitled Document

Look at the examples of some common statements about geometric figures and the supporting reasons.

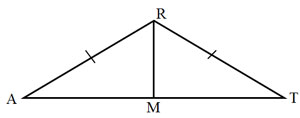
Based on the figure below and that M is the midpoint of AT, we can say that that ΔARM≅ΔTRM. Why can we say this?

Choose from the facts, definitions, postulates, or theorems below, to justify the statement.

shows isosceles triangle ART with median RM and AR congruent to RT

RM≅RM	Interactive button. Assistance may be required. Check Your Answer Correct, a segment is congruent to itself.
AM = ½AT	Interactive button. Assistance may be required. Check Your Answer This is a correct statement but does not help to justify the statement.
AM≅MT	Interactive button. Assistance may be required. Check Your Answer Correct, this names a pair of congruent sides.
SAS	Interactive button. Assistance may be required. Check Your Answer Incorrect because we haven't shown any congruent angles.
AAS	Interactive button. Assistance may be required. Check Your Answer Incorrect because we haven't shown any congruent angles.
SSS	Interactive button. Assistance may be required. Check Your Answer Yes, we have 3 pairs of congruent sides.

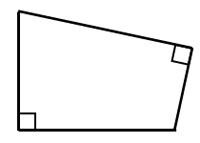
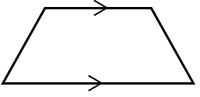
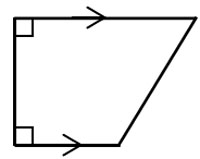
Based on the information below, what polygon is being described?

Choose the figure which best fits the description.

	Interactive popup. Assistance may be required. Check Your Answer Incorrect, right angles are not consecutive.
	Interactive popup. Assistance may be required. Check Your Answer Incorrect, there are no right angles.
	Interactive popup. Assistance may be required. Check Your Answer Correct.
	Interactive popup. Assistance may be required. Check Your Answer Incorrect, there are two pairs of parallel sides.

Click on the links below to reveal some of the most commonly used justifications for geometry proofs.

Congruent Triangles Some common reasons used to justify statements about congruent triangles are shown.	Interactive popup. Assistance may be required. Common Justifications Side-Side-Side Postulate Side-Angle-Side Postulate Angle-Side-Angle Postulate Angle-Angle-Side Theorem Corresponding parts of congruent triangles are congruent
Quadrilaterals Some common reasons used to justify statements about quadrilaterals are shown.	Interactive popup. Assistance may be required. Common Justifications Opposite sides of a parallelogram are congruent Opposite angles of a parallelogram are congruent Diagonals of a rectangle bisect each other Diagonals of a rhombus are perpendicular A rectangle is a parallelogram with 4 right angles.
Parallel and Perpendicular Lines Some common reasons used to justify statements about parallel and perpendicular lines are shown.	Interactive popup. Assistance may be required. Common Justifications Corresponding Angles Postulate Alternate Interior Angles Theorem Alternate Exterior Angles Theorem Consecutive Interior Angles Theorem Perpendicular Transversal Theorem
Similar Polygons Some common reasons used to justify statements about similar polygons are shown.	Interactive popup. Assistance may be required. Common Justifications Angle-Angle Similarity Postulate Side-Side-Side Similarity Theorem Side-Angle-Side Similarity Theorem Definition of similar figures

Sometimes, logical reasoning is used just for fun. In this case, you can describe how you arrived at your conjecture or solution very informally.

A basket contains five apples. Do you know how to divide them among five kids so that each one has an apple and one apple stays in the basket?

Interactive popup. Assistance may be required.

Kids get an apple (one apple for each one of them) and the fifth kid gets an apple with the basket still containing the apple.