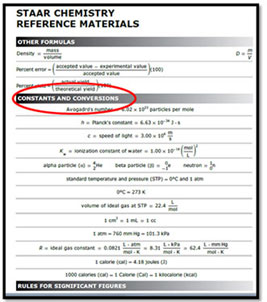
Source: STAAR Reference Material, Texas Education Agency
When you are performing mole conversion problems, it is important to remember how to perform dimensional analysis and the rules for significant figures. Often, you will need to express your answers in scientific notation. Let’s briefly review each of these skills.
Remember the STAAR reference material for Chemistry has a section titled Constants and Conversions. Refer to this as you work various problems.

Source: STAAR Reference Material, Texas Education Agency
Listed below are some other common unit conversions as well as common metric prefixes used in science.

Write the given information as a fraction by placing it over 1. (Placing it over 1 makes it a fraction but does not change its value.)

The STAAR reference material for Chemistry document lists the rules for significant figure in a section titled Rules For Significant Figures.
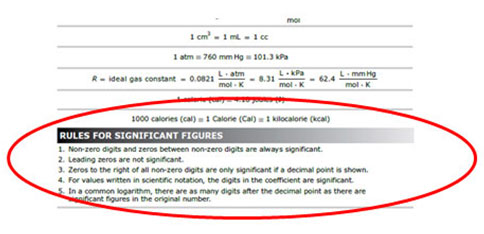
Source: STAAR Reference Material, Texas Education Agency
The rule for addition and subtraction with significant figures is as follows:
When measurements are added or subtracted, the final answer can contain no more decimal places than the least accurate measurement.
When adding and subtracting measurements, the level of accuracy at which you express your final answer does not depend on the number of significant figures in the original problem but instead is determined by the position or place value of the least significant digit in the original problem.
The rule for multiplication and division with significant figures is as follows:
When measurements are multiplied or divided, the answer can contain no more significant figures than the least accurate measurement.
This rule simply means the final answer can be no more accurate than the least accurate measurement. Count the significant figures in each measurement instead of the number of decimal places when multiplying and dividing measurements.