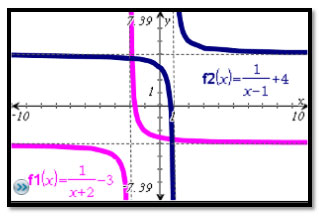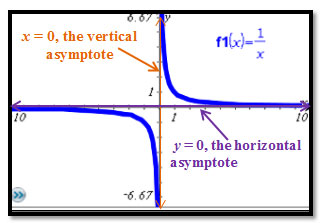
The parent rational function, f(x) = 1 over x 1 x , has a vertical asymptote at x = 0 and a horizontal asymptote at y = 0. Changing the parameters a, h, and k, will change the intersection point of the asymptotes. This point will help you when describing the transformation that has occurred.

What happens to the graph of a rational function when you change the parameters of a, h, and k?
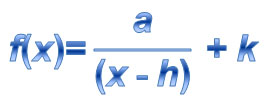

![]() In the interactive below, set b = 1. Begin with only changing the value for a, leaving h and k equal to zero. Then, change only the value of h, leaving a = 1 and k = 0. Finally, change only the value of k, leaving a = 1 and h = 0.
In the interactive below, set b = 1. Begin with only changing the value for a, leaving h and k equal to zero. Then, change only the value of h, leaving a = 1 and k = 0. Finally, change only the value of k, leaving a = 1 and h = 0.
Interactive popup. Assistance may be required.
As the value of a increased (a > 1), the graph was vertically stretched.
Interactive popup. Assistance may be required.
As the value of a got closer to 0, the graph was vertically compressed.
Interactive popup. Assistance may be required.
When the value of a became negative, the graph reflected across the horizontal asymptote.
Interactive popup. Assistance may be required.
As the value of h increased, the function shifted to the right. As the value of h decreased, the function shifted to the left.
Interactive popup. Assistance may be required.
As the value of k increased, the function shifted up. As the value of k decreased, the function shifted down.
Interactive popup. Assistance may be required.
The vertical asymptote intersects the x-axis at the value of h, and the horizontal asymptote intersects the y-axis at the value of k.
How were the methods used in this lesson to transform the rational function similar to those used to transform other types of functions, such as quadratics or exponential functions?

Now think about the square root function f(x) = a√(x – h) + k. What do you predict will happen when changes are made to a, h, and k?
Interactive popup. Assistance may be required.
The change is in a, and its value is greater than 1.
Interactive popup. Assistance may be required.
The graph of the parent function is vertically stretched by a factor of 3.
Interactive popup. Assistance may be required.
Look for the constant, k, being added or subtracted to the parent function, f(x) = 1 over x 1 x .
Interactive popup. Assistance may be required.
A horizontal shift is a change in h. If the vertical asymptote is originally at x = 3 and you moved it to the right 10 units, the new vertical asymptote would be at x = 3 + 10.