Each conic section has its own equation in standard form and is a form of the general equation for conic sections. You will study those specific equations in a different lesson. The general form of the equation for conic sections is:

Ax2 + Bxy + Cy2 + Dx + Ey + F = 0

General form is the same for all of the conic sections. The values of the coefficients determine the type of conic section. For this section, we will let B = 0.

Interpreting the Parameters A and C

Below is a screen shot and link to an online applet. Click on the image to launch a new window/tab and follow the directions below. When you are done with this exercise, close the window/tab to return to this lesson.

This activity might not be viewable on your mobile device.Interactive exercise. Assistance may be required.

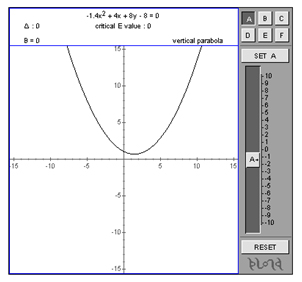
  1. Use the slider to set the value for B to zero.
  2. Use the sliders to set the values for A and C as shown in the table below.
  3. In your notes, create and complete a table like the one shown below. The first one is done for you.

    4. Interactive exercise. Assistance may be required.

  4. After you complete your table, mouse over the table above to check your answers.
  5. Answer the questions below.

Summarize Your Findings

  1. When A·C was positive, what type of conic section was generated?
    2. Interactive popup. Assistance may be required.

    Check Your Answer

    circle or ellipse Close Pop Up
  2. When A·C was positive and A = C, what type of conic section was generated?
    3. Interactive popup. Assistance may be required.

    Check Your Answer

    circle Close Pop Up
  3. When A·C was negative, what type of conic section was generated?
    4. Interactive popup. Assistance may be required.

    Check Your Answer

    hyperbola Close Pop Up
  4. When A·C was zero and both A and C were not zero, what type of conic section was generated?
    5. Interactive popup. Assistance may be required.

    Check Your Answer

    parabola Close Pop Up
  5. When A·C was zero, and both A and C were zero, what type of conic section was generated?
    6. Interactive popup. Assistance may be required.

    Check Your Answer

    line Close Pop Up
Generalize

For the general form equation Ax2 + Bxy + Cy2 + Dx + Ey + F = 0, and B = 0, the equation generates:

  • an ellipse if
  • a circle if
  • a hyperbola if
  • a parabola if
  • a line if
  1. A · C > 0 and A ≠ C
  2. A · C > 0 and A = C
  3. A · C < 0
  4. A · C = 0 and eitherA = 0 or C = 0
  5. A · C = 0 and bothA = 0 and C = 0