This section explores how the changes a affect the exponential equation y = ab^x.

The change in the variable affects the graph of the function.

Using the applet, create the same graph shown in the image below.

Exponential Equations

This is a screenshot of the applet.

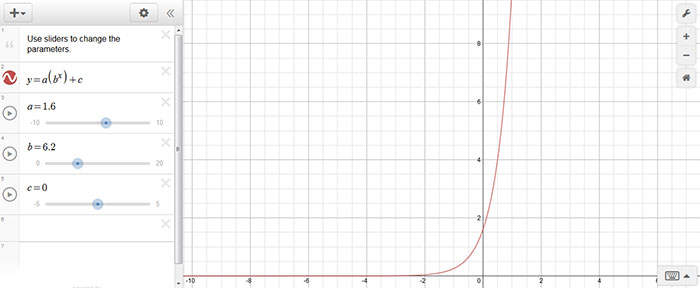
Source: Analyze Math, Exponential Functions

Answer the following in your notes.

Click on the blanks to reveal the answers.

• The exponential equation is Interactive button. Assistance may be required. ____ y = 2^x .
• In the lower left hand corner, change the B to 0.5, the new equation is Interactive button. Assistance may be required. ____ y = 0.5^x the a–value is Interactive button. Assistance may be required. ____ 1 .
• The equation is an exponential Interactive button. Assistance may be required. ____ decay function with a y-intercept of Interactive button. Assistance may be required. ____ 1 and the y-values are Interactive button. Assistance may be required. ____ halved as the x-values are increased.
• In the upper left hand corner, change a to 2, the new equation is Interactive button. Assistance may be required. ____ y = 2(0.5)^x and the y–intercept is Interactive button. Assistance may be required. ____ 2 .
• Change a to 3, the new equation is Interactive button. Assistance may be required. ____ y = 3(0.5)^x and the y–intercept is Interactive button. Assistance may be required. ____ 3 .
• When a increases from 2 to 3, the y–intercept Interactive button. Assistance may be required. ____ increases, the a-value is the y–intercept while the slope becomes Interactive button. Assistance may be required. ____ steeper.
• Change a to 1, the new equation is Interactive button. Assistance may be required. ____ y= 0.5^x and the y–intercept Interactive button. Assistance may be required. ____ 1.
• Change a to -1, the new equation is Interactive button. Assistance may be required. ____ y= -1(0.5)^x and the y–intercept is Interactive button. Assistance may be required. ____ -1
• The equation y = -(0.5)^x is a Interactive button. Assistance may be required. ____ reflection of the equation y = 2^x over the x–axis.

Experiment with other variables.