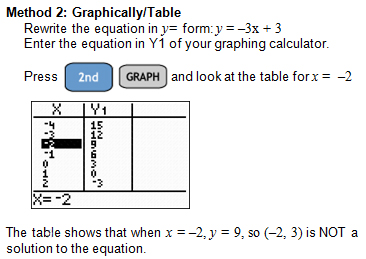
In certain instances you are asked to determine if a certain point is a solution to a particular linear equation.

For example: Is (-2, 3) a solution to the equation 3x + y = 3?

There are two different ways to determine if the given point is a solution to the given equation.

Method 1: Algebraically

Substitute the given values for x and y into the equation.

3x + y = 3
3(-2) + 3 = 3
-6 + 3 ≠ 3
-3 ≠ 3

Since the equation is false, we know that (-2, 3) is NOT a a solution to the equation.

Graphing calculator image and text: Rewrite the equation in y= form: y = –3x + 3 Enter the equation in Y1 of your graphing calculator. Press '2nd' 'Graph' and look at the table for x = –2 The table shows that when x = –2, y = 9, so (–2, 3) is NOT a solution to the equation.

Practice: Use the graphing calculator link below to determine if the given points are solutions to the given equations. Write the answers on your own paper.

This activity might not be viewable on your mobile device. Interactive exercise. Assistance may be required.Equation Grapher

Directions

  1. Is (-3, -5) a solution for y = 2x + 1?
  2. Is (2, -2) a solution for 4x – 2y = 10?
  3. Is (-3, -3) a solution for 6x – 3y = -9?
Interactive popup. Assistance may be required.

Check Your Answers

  1. Yes: -5 = 2(-3) + 1 → -5 = -6 + 1 → -5 = -5
  2. No: 4(2) – 2(-2) = 10 → 8 + 4 ≠ 10 → 12 ≠ 10
  3. Yes: 6(-3) – 3(-3) = -9 → -18 + 9 = -9 → -9 = -9
Close Pop Up

More Practice

On your own paper, determine if the given point is a solution to the given equation algebraically.

  1. Is (-3, -5) a solution for y = 3x + 1?
  2. Is (3, 1) a solution for 4x – 2y = 10?
Interactive popup. Assistance may be required.

Check Your Answers

  1. No: -5 = 3(-3) + 1 → -5 ≠ -9 + 1 → -5 ≠ -8
  2. Yes: 4(3) – 2(1) = 10 → 12 - 2 = 10 → 10 = 10
Close Pop Up