
Source: Birthday Cake, Will Clayton, Flickr
This resource will compare linear and non-linear functions.
A linear function has a constant rate of change or slope. To find the slope, information from the problem situation can be put into a table, and the slope can be calculated.

Source: Birthday Cake, Will Clayton, Flickr
Joey wants to have his birthday party at Super Cool Pizza. To reserve the party room, it costs $85 plus $6 per guest.

Source: Pizza sliced up, VancityAllie, Flickr
To determine this is linear relationship, a table of values can be calculated using the information in the problem.
| Number of Friends | Cost of Party | |
|---|---|---|
| The party will cost $85 before he invites any friends. | 0 | $85 |
| If Joey invites one friend, the party will cost $91. | 1 | $91 |
| If Joey invites two friends, the party will cost $97. | 2 | $97 |
| For each friend Joey invites, the cost is increased by $6. | 3 | $103 |
The slope can be calculated by looking at the changes in the values from the table.
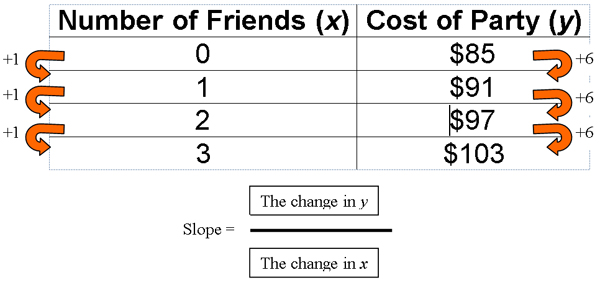
The y values in the table are increasing by $6, the x values are increasing by one friend. The slope is the change in y divided by the change in x, 6 1 or 6. Since the change in the y values and the change in the x values is constant, it stays the same. The function is linear.
If y represents the cost of the party, this expression (6 times x plus 85) can be written as an equation equal to the cost of the party.
6(x) + 85
y = 6(x) + 85
This is a linear equation because the rate of change ($6 per friend) remains the same throughout the table.