Find the value of y when x = -4 for the square root function graphed below.

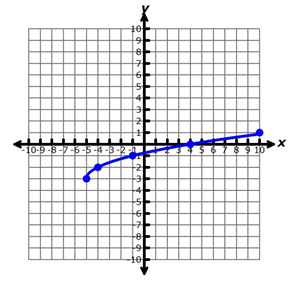
A. y = -4
Incorrect. y = −4 is not in the range for this graph.

B. y = -2
Correct!

C. y = 0
Incorrect. The point (−4, 0) is not on the graph.

D. y = 1
Incorrect. The point (−4, 1) is not on the graph.

The graph of the square root function, y = -√x − 1 + 2, is shown below.

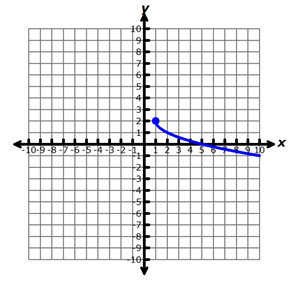
According to this graph, what is the solution to the equation -√x − 1 + 2 = 1?

A. x = 1
Incorrect. When x = 1, the y-value is 2, which does not agree with the right side of the equation.

B. x = 2
Correct!

C. x = 5
Incorrect. This number is the x-coordinate of the x-intercept, which would be the solution to the equation -√x − 1 + 2 = 0.

D. x = 0
Incorrect. 0 is not in the domain of the function, so it cannot be a solution to a related equation.

The table below shows some values of the equation .

According to the table, what is the solution to the equation ?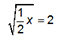

A. x = 1
Incorrect. When the x = 2, y = 1, but in the equation, y = 2.

B. x = 8
Correct!

C. x = 18
Incorrect. Look at the table again and find the x-value when y = 2.

D. You cannot determine this value from this table.
Incorrect. The value of x when y = 2 is shown in the table.

The speed in miles per hour, s, of a tsunami can be modeled by the function s(d) = 4.2√d, where d is the average depth in feet of the water over which the tsunami travels. Use the graph to estimate the depth of water for a tsunami traveling 100 miles per hour.

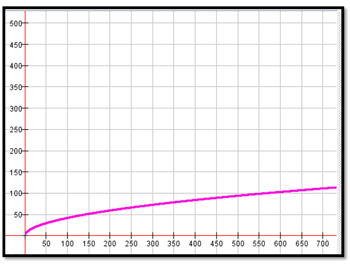
A. 550 feet
Correct!

B. 400 feet
Incorrect. Look at the graph again when s(d) = 100.

C. 300 feet
Incorrect. Look at the graph again when s(d) = 100.

D. 45 feet
Incorrect. This value represents the speed of a tsunami traveling through water with a depth of 100 feet.

The side length, s, of a cube can be modeled by the square root function 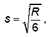where R represents the surface area of the cube. Use the table below to determine the side length of a cube with a surface area of 54 square units.

A. 0 units
Incorrect. Look at the table again to find s when R = 54.

B. 1 unit
Incorrect. Look at the table again to find s when R = 54.

C. 2 units
Incorrect. Look at the table again to find s when R = 54.

D. 3 units
Correct!