The formula C = 5 9 (F − 32) gives the degrees Celsius as a function of the degrees Fahrenheit. You would like to convert 16° Celsius to Fahrenheit  using the inverse of the function C = 5 9 (F – 32). Find the inverse of the function and the degrees Celsius.

A. F = 5 9 (C – 32), -8.89° F
Incorrect. Find the inverse of the function by solving for F.

B. F = 5 9 (C + 32), 40.89° F
Incorrect. Find the inverse of the function by solving for F.

C. F = 9 5 (C + 32), 60.8° F
Correct!

D. F = 9 5 (C + 32), 86.4° F
Incorrect. Find the inverse on the function by solving for F.

Which of the following does NOT represent a function and its inverse?

A.

f(x)
X -2 0 4
Y 3 -2 -8

f -1(x)
X -8 -2 3
Y 4 0 -2

Incorrect. These two tables do correctly represent a function and its inverse. Look at the correlation between the x and y values for f(x) and the inverse function.

B.

Incorrect. This graph correctly represents a function and its inverse. Notice that the two lines reflect over y=x.

C.
f(x) = 3x + 6
f -1(x) = 1 3 x − 2
Incorrect. These equations do represent a function and its inverse. You can check by substituting 3x + 6 in for x of the inverse and see if you get x.

D.

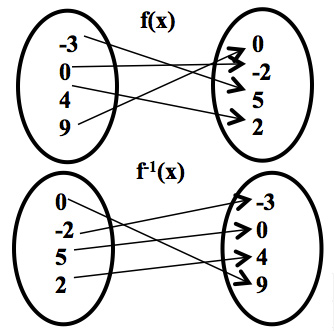
Correct! These mapping diagrams do NOT represent a function and its inverse. Remember that an inverse function interchanges the input and output values.

Maria is making tacos for a friend’s birthday party. She decided it would be easier to make a table showing the number of tacos needed depending on the number of people attending. Below is the table Maria created.

People
Tacos
2
5
5
12
8
20
12
32
15
40

The host wasn’t sure how many people to invite, so Maria showed her the inverse of the table she created. Determine the inverse of this table.

A.

Tacos
People
2
5
5
12
8
20
12
32
15
40

Incorrect. This table is exactly the same as the original table.

B.

Tacos
People
40
15
32
12
20
8
12
5
5
5

Correct!

C.

Tacos
People
40
2
32
5
20
8
12
12
5
15

Incorrect. Exchange the x’s and y’s in the original table.

D.

Tacos
People
2
40
5
32
8
20
12
12
15
5

Incorrect. Exchange the x’s and y’s in the original table.

Which of the following graphs correctly shows a function and its inverse?

A.

Incorrect.The graphs do not reflect over y=x.

B.

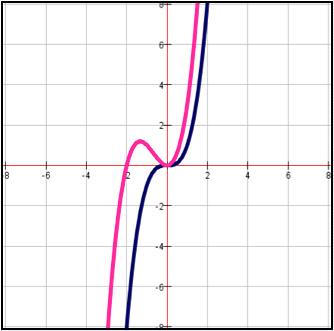
Incorrect.The graphs do not reflect over y=x.

C.

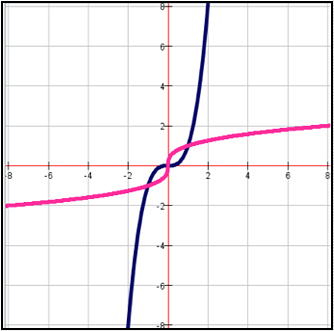
Correct! The graphs reflect over y = x.

D.

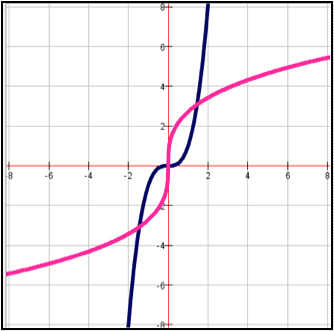
Incorrect.While it’s close, the graphs do not reflect over y = x.

Saul was practicing finding the inverse of functions several different ways. Once he found the inverse of the function, he was trying to decide which inverse was a function or an inverse function. Select the function that has an inverse function or a one-to-one correspondence.

A.

x
y
-2
3
0
-4
1
3
2
0
3
2

Incorrect. Create a table showing the inverse of the function. Notice in the table that 3 has two possible coordinates.

B. f(x) = 2x2 – 3.5
Incorrect. The inverse of the function is which is not a function; you can sketch it and use the vertical line test to double check.

C.

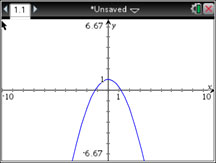
Incorrect. Sketch the inverse of the function reflecting over the line y = x and then use the vertical line test to see if the inverse is a function.

D.

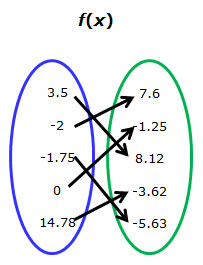
Correct!