Question 1: Which statement correctly describes the effect on the graph of y = -2 (x – 1)2 + 3 when it is changed to y = ½ (x – 1)2 + 3?
|
Answer Choice |
Response |
A |
The graph is wider and opens upward. |
Correct! ½ is less than |-2| which will result in a wider parabola. It will also open upward because ½ is positive. |
B |
The graph is wider and opens downward. |
Incorrect: "a" is positive, so the graph opens upward. |
C |
The graph is narrower and opens upward. |
Incorrect. It does open up, but for the parabola to be narrower |a|>1 |
D |
The graph is narrower and opens downward |
Incorrect. For the parabola to be narrower and open down … a must be negative and |a|>1. |
Question 2: Which statement correctly describes the effect on the graph of f(x) = 3 (x + 7)2 + 4 when it is changed to f(x) = 3 (x + 7)2 − 1?
|
Answer Choice |
Response |
A |
The graph is translated 5 units to the right. |
Incorrect. When “k” is changed it is a vertical translation. |
B |
The graph is translated 5 units to the left. |
Incorrect. When “k” is changed it is a vertical translation. |
C |
The graph is translated 5 units down. |
Correct! The change in “k” from 4 down to -1 is a difference in 5 units. |
D |
The graph is translated 5 units up. |
Incorrect. “k” changes from 4 to –1, so the parabola moves down. |
Question 3: Which statement correctly describes the effect on the graph of y = 2 (x – 5)2 - 3 when it is changed to y = 2 (x + 2)2 - 3?
|
Answer Choice |
Response |
A |
The graph is translated 7 units to the right. |
Incorrect. Since “h” changes from 5 to -2, it would be a translation to the left. |
B |
The graph is translated 7 units to the left. |
Correct! The difference between 5 and -2 is 7 units and it would translate to the left. |
C |
The graph is translated 7 units down. |
Incorrect. When “h” is changed it is a horizontal translation. |
D |
The graph is translated 7 units up. |
Incorrect. When “h” is changed it is a horizontal translation. |
Question 4: Which statement correctly describes the graph of y = -4(x + 3)2 - 1?
|
Answer Choice |
Response |
A |
It is narrower than the graph of y = x2 translated 3 units to the left and 1 unit up |
Incorrect. “k” = -1. The negative will translate the graph down not up. |
B |
It is wider than the graph of y = x2, reflected across the x-axis, then translated 3 units to the right and 1 unit down |
Incorrect. For a parabola to be wider than y=x2, |a|<1. |
C |
It is the graph of y = x2, reflected across the |
Incorrect. “k” = -1. The negative will translate the graph down not up. |
D |
It is narrower than the graph of y = x2, reflected across the x-axis, then translated 3 units to the left and 1 unit down. |
Correct! Since |-4| > 1, the graph will be narrower. Since “a” = -4, the negative will reflect the graph across the x-axis, and since “k” = -1, the graph will translate down 1 unit. |
Question 5: The graph of f(x) = -5 (x - 1)2 + 7 is translated 3 units to the right. What is the equation of the new graph?
|
Answer Choice |
Response |
A |
f(x) = -5 (x - 4)2 + 7 |
Correct! 1 + 3 = 4 so the new “h” would equal 4. |
B |
f(x) = -5 (x + 2)2 + 7 |
Incorrect. This equation is translated 3 units to the left. |
C |
f(x) = -5 (x - 1)2 + 10 |
Incorrect: Remember that a horizontal translation affects the “h” in the equation f(x) = a (x - h)2 + k |
D |
f(x) = -2 (x - 1)2 + 7 |
Incorrect. Remember that a horizontal translation affects the “h” in the equation f(x) = a (x - h)2 + k |
Question 6: Which of the following functions is a transformation of y = x2, where the new parabola is wider and has been translated to the left?
|
Answer Choice |
Response |
A |
y = ⅝ (x - 7)2 |
Incorrect. This one is wider than y = x2, but is translated to the right instead of to the left. |
B |
y = ½ x2 − 6 |
Incorrect. This one is wider than y = x2, but is translated down instead of to the left. |
C |
y = 4 (x + 5)2 |
Incorrect. This one is translated to the left, but is narrower than y = x2 |
D |
y = ¾ (x + 3)2 |
Correct! This one is wider and has been translated to the left. |
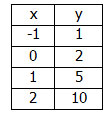
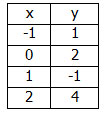
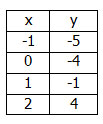
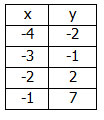
Question 7: You have been given a table of values for the function y =(x + 1)2 – 2.

If the graph of this function is translated 3 units down, which of the tables shown below would be points on the new parabola?
A.  |
B. |
C.  |
D.  |
A. Incorrect. You added 3 instead of subtracting 3 from the y values.
B. Incorrect. You should subtract 3 from ALL the y values.
C. Correct! Subtracting 3 from the y values will give you these correct values in the table.
D. Incorrect. You subtracted 3 from the x values instead of the y values.