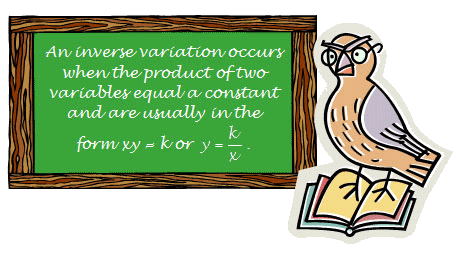
In this section, tables are analyzed to determine if they represent an inverse variation.

The table below represents a relationship that is an inverse variation. Multiply the values for x and y together in each column. Use the link beneath the table to check your answer.

Example

x	2	3	4
y	6	4	3
Process	2 × 6 = ___	3 × 4 = ___	4 × 3 = ___

Interactive popup. Assistance may be required. Check Your Answer

2×6=12
3×4=12
4×3=12

• What do you notice about the products xy for each pair of numbers?

  Interactive popup. Assistance may be required.

  Check Your Answer

  The products are the same, and they are 12 each time.

The table below represents a relationship that is NOT an inverse variation. Multiply the values for x and y together in each column. Use the link beneath the table to check your answer.

x	2	3	4
y	4	6	8
Process	2 × 4 = ___	3 × 6 = ___	4 × 8 = ___

Check Your Answer

2×4=8
3×6=18
4×8=32

• What do you notice about the products xy for each pair of numbers?

  Interactive popup. Assistance may be required.

  Check Your Answer

  The products are different.

Pause and Reflect

If a relationship between x and y is an inverse variation, what can you say about the product of each pair of numbers xy?

Interactive popup. Assistance may be required.

Check Your Answer

If a relationship is an inverse variation, the product xy will be the same for each pair of numbers x and y.

A constant product for an inverse variation is also called the constant of proportionality, k. How are k and the product xy related?

Interactive popup. Assistance may be required.

Check Your Answer

xy = k.