
Incorrect. The roots of a function can be found by finding the x-intercepts (when y = 0). To determine if these are the correct roots, substitute the values in the function and check.
Given the table below, estimate the roots of the function f(x) = x2 − 2x - 4.
x
f(x)
-3
11
-2
4
-1
-1
0
-4
1
-5
2
-4
3
-1
4
4
5
11
A. The roots of this function will lie between the x values of 0 and 1 and between the x values of 4 and 5.
Incorrect. To estimate the roots given a table, find where the y value changes from negative to positive and positive to negative. The roots will lie between the corresponding x values where these changes take place.
B. The roots of this function will lie between the x values of -2 and -1 and between the x values of 0 and 1.
Incorrect. To estimate the roots given a table, find where the y value changes from negative to positive and positive to negative. The roots will lie between the corresponding x values where these changes take place.
C. This function does not have any roots.
Incorrect. To estimate the roots given a table, find where the y value changes from negative to positive and positive to negative. The roots will lie between the corresponding x values where these changes take place.
D. The roots of this function will lie between the x values of -2 and -1 and between the x values of 3 and 4.
Correct! The roots of this function lie between the x values of -2 and -1 and between 3 and 4.
Given the table below, determine the roots of the function f(m) = m2 − 5m − 14 = 0.
m
f(m)
-3
10
-2
0
-1
-8
0
-14
5
-14
6
-8
7
0
A. The roots of the function are (-2, 0) and (0, -14).
Incorrect. The roots of a function can be found by finding the x-intercepts (when y = 0).
B. The roots of the function are (-2, 0) and (7, 0).
Correct! The roots of the function are (-2, 0) and (7, 0). The roots are the x-intercepts of the function.
C. The roots of the function are (0, -14) and (5, -14).
Incorrect. The roots of a function can be found by finding the x-intercepts (when y = 0).
D. The roots of the function are (0, -2) and (0, 7).
Incorrect. The roots of a function can be found by finding the x-intercepts (when y = 0).
Which table shows the roots of the function f(x) = x2 − 9x + 20?
A.

Incorrect. The roots of a function can be found by finding the x-intercepts (when y = 0). To determine if these are the correct roots, substitute the values in the function and check.
B.

Correct! This table shows the correct roots of the function. The roots are the x-intercepts or when y = 0.
C.
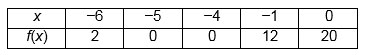
Incorrect. The roots of a function can be found by finding the x-intercepts (when y = 0). To determine if these are the correct roots, substitute the values in the function and check.
D.
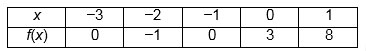
Incorrect. The roots of a function can be found by finding the x-intercepts (when y = 0). To determine if these are the correct roots, substitute the values in the function and check.
Given the table below, determine the roots to the function f(a) = a2 + 6a − 7.
a
f(a)
-8
9
-7
0
-2
-1
0
-7
1
0
3
20
8
105
A. The roots of the function are (-7, 0) and (1, 0).
Correct! The roots of the function are (-7, 0) and (1, 0). The roots are the x-intercepts of the function.
B. The roots of the function are (-8, 9) and (8, 105).
Incorrect. The roots of a function can be found by finding the x-intercepts (when y = 0).
C. The roots of the function are (-7, 0) and (0, -7).
Incorrect. The roots of a function can be found by finding the x-intercepts (when y = 0).
D. The roots of the function are (0, -7) and (0, 1).
Incorrect. The roots of a function can be found by finding the x-intercepts (when y = 0).
The table below contains the function values for given x-values of a quadratic function, f(x).
|
x
|
f(x)
|
|
-6
|
18
|
|
-4
|
0
|
|
-2
|
-15
|
|
0
|
-12
|
|
2
|
-6
|
|
3
|
0
|
|
4
|
8
|
Which of the following functions best represents the function whose solutions to the equation f(x) = 0 are shown in the table?
A. f(x) = x2 + x – 12
Correct!
B. f(x) = x2 – x – 12
Incorrect. This function has the same y-intercept as the function represented by the table values, but the solutions (x-intercepts) are different.
C. f(x) = x2 + 3x – 4
Incorrect. The solutions to the equation f(x) = 0 are represented by the points in the table where f(x) = 0.
D. f(x) = x2 – 3x + 4
Incorrect. The solutions to the equation f(x) = 0 are represented by the points in the table where f(x) = 0.