1. Which expression represents the area of a rectangle with sides measuring x²y and 7xy²?

A. 7x³y³	Correct! The exponents of the x-variables and the y-variables were added.
B. 7x²y²	Incorrect. Find the sum of the exponents of the x-variables and the y-variables.
C. 7x⁴y²	Incorrect. Check the addition of the exponents of the x-variables.
D. 7x³y⁴	Incorrect. Check the addition of the exponents of the y-variables.

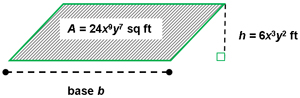
2. The area, A, of a banner shaped like a parallelogram is 24x⁹y⁷ square feet. The height, h, of the banner is 6x³y² feet. The area of a banner can be found by using the formula A = bh. Which of the following best represents the length of this banner's base, b?

A. 6x⁶y⁵ feet	Incorrect. To find the base divide the area by the height remembering to subtract the exponents of the x and the y-variables.
B. 6x¹²y⁹ feet	Incorrect. Incorrect. To find the base divide the area by the height remembering to subtract the exponents of the x and the y-variables.
C. 4x⁶y⁵ feet	Correct! The base was found by dividing the area by the height which involved subtracting the exponents of the x and the y-variables.
D. 4x¹²y⁹ feet	Incorrect. To find the base divide the area by the height remembering to subtract the exponents of the x and y-variables.

3. If 3m^-2n⁴ represents the length of a tile floor and 2m⁶n^-7 represents the width of the tile floor. Find the area of the tile floor using the formula. A = bh.

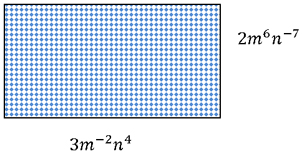
A. 6m⁴n³	Incorrect. The exponents were added but a negative exponent is converted to a reciprocal.
B. 6 m⁴n³	Incorrect. The exponents were added correctly but the m-variable wasn’t negative and didn’t need to be changed to a reciprocal.
C. 6n³ m⁴	Incorrect. The exponents were added incorrectly.
D. 6m⁴ n³	Correct! The exponents were added correctly and the negative exponent was converted to the reciprocal.

4. Susan’s total business income per month is represented by the expression r⁴s³t². The total products manufactured are represented by r⁰st⁰. To find her monthly income per item, simplify the expression r⁴s³t² r²st⁰

A. r²s²t	Incorrect. The exponents of the t-variable were not subtracted correctly, 2 − 0 = 2
B. r²s²t²	Correct. The exponents were subtracted correctly.
C. r²s²t³	Incorrect. The exponents of the s-variable were not subtracted correctly, 3 − 1 = 2
D. r⁴s²t³	Incorrect. The exponents of the r-variable were not subtracted correctly, 4 − 2 = 2

5. In order to estimate his expenses for his shoe shop, Jason needs to square the expression, (m⁴n^-2o²)

Simplify (m⁴n^-2o³)².

A. m⁸o⁴ n⁴	Correct! The exponents were multiplied and the negative exponents were converted to positive by using the reciprocal.
B. m⁸n⁴ o⁴	Incorrect. The exponents were multiplied but the wrong variable was converted to a reciprocal.
C. m⁴o⁴ n⁴	Incorrect. The m-variable was multiplied incorrectly.
D. m⁸o² n⁴	Incorrect. The o-variable was multiplied incorrectly.