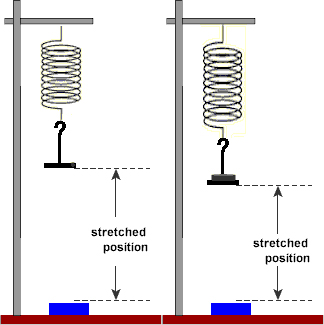
Source: Double springs simulating Hooke's Law, Catharine H. Cowell, physicslab.org
Selena earns $40.50 per hour. In addition, she receives a monthly stipend of $125 for expenses. The numbers of hours Selena worked per month and her monthly income for the past year are recorded in the table below.
| Month | Numbers of Hours Worked Per Month x | Monthly Income y |
|---|---|---|
| January | 160 | 6605 |
| February | 150 | 6200 |
| March | 160 | 6605 |
| April | 120 | 4985 |
| May | 110 | 4580 |
| June | 160 | 6605 |
| July | 150 | 6200 |
| August | 160 | 6605 |
| September | 120 | 4985 |
| October | 110 | 4580 |
| November | 90 | 3770 |
| December | 90 | 3770 |
Does the data in the table represent a functional relationship? If so, what is the function rule?
A. No. A functional relationship does not exist and there is no function rule.
Incorrect. In a functional relationship, there is exactly one output value for every input value. Review the information in the table to determine if a function rule exists.
B. Yes. The data represents a functional relationship but there is no function rule.
Incorrect. Review the information in the table to determine if a function rule exists.
C. Yes. The data represents a functional relationship and there is a function rule. The function rule is y = 40.50x + 125.
Correct! In a functional relationship, there is exactly one output value for every input value and a function rule does exist.
D. Yes. The data represents a functional relationship and there is a function rule. The function rule is y = 40.50x + 1500.
Incorrect. Review the information in the table to determine the correct function rule.
Last week, Mrs. Kerley's physics classes studied Hooke's Law – a basic law in elasticity. Hooke's Law states that there is a relationship between the amount of force applied to a spring and the length the spring stretches as a result. An experiment using a particular spring yielded the following results.

Source: Double springs simulating Hooke's Law, Catharine H. Cowell, physicslab.org
| X Distance in meters | F Force in Newtons |
|---|---|
| 0.2 | 2 |
| 0.3 | 3 |
| 0.8 | 8 |
| 1.1 | 11 |
Does the data in the table represent a functional relationship? If so, what is the function rule?
A. No. The data does not represent a functional relationship and there is no function rule.
Incorrect. In a functional relationship, there is exactly one output value for every input value. Review the information in the table to determine if a function rule exists.
B. Yes. The data represents a functional relationship, but a function rule does not exist.
Incorrect. In a functional relationship, there is exactly one output value for every input value. Review the information in the table to determine if a function rule exists.
C. Yes. The data represents a functional relationship and there is a function rule. The function rule is f = 10x.
Correct! There is exactly one output value for every input value and a functional rule does exist.
D. Yes. The data represents a functional relationship and there is a function rule. The rule is F = 0.1x.
Incorrect. Yes the data represents a function however the function rule is incorrect. Recall that F is the dependent quantity in this experiment.
Race More Gas Station, located in south Texas, generated a report for the month of February to analyze the price per gallon for regular gas. The collected data is shown in the table below.
| Week Number x | Price per gallon y |
|---|---|
| Week 1 | $3.60 |
| Week 2 | $3.70 |
| Week 2 | $3.75 |
| Week 3 | $3.82 |
| Week 4 | $3.86 |
Does this data represent a functional relationship?
A. Yes, because there is exactly 1 price for each week.
Incorrect. In a functional relationship, there is exactly one output value for every input value.
B. No, because there are 2 prices for week 2.
Correct! In a functional relationship, there is exactly one output value for every input value and a function rule does exist.
C. Yes, because the price per gallon does not decrease.
Incorrect. In a functional relationship, there is exactly one output value for every input value.
D. No, because there should be another price for week 1.
Incorrect. All of the information is given in the table.
The gravitational weights on Earth, x, of several persons and their gravitational weights on the Moon, y, are given in the table below.
| Gravitational Weight on Earth x | Gravitational Weight on Moon y |
|---|---|
| 120 | 19.9 |
| 130 | 21.5 |
| 170 | 28.2 |
| 130 | 21.5 |
Does this data represent a functional relationship?
A. Yes, because there is exactly 1 gravitational weight on the Moon for every gravitational weight on Earth.
Correct! In a functional relationship, there is exactly one output value for every input value.
B. No, because there are two gravitational weights on the moon for the gravitational weight of 130 on Earth.
Incorrect. In a functional relationship, there is exactly one output value for every input value.
C. No, because a person should weigh more on the moon.
Incorrect. In a functional relationship, there is exactly one output value for every input value.
D. No, The moon does not have gravity.
Incorrect. All of the information is given in the table.
This week, Bonnie was offered a job with an annual salary of $30,000 plus a $500 bonus every quarter. Her potential earnings per quarter are shown in the table below.
Does this information represent a functional relationship? If so, what is the function rule?
| Quarter x | Earnings y |
|---|---|
| 1 | $30,500 |
| 2 | $31,000 |
| 3 | $31,500 |
| 4 | $32,000 |
A. No. The data does not represent a functional relationship and a function rule does not exist.
Incorrect. In a functional relationship, there is exactly one output value for every input value. Review the information in the table to determine if a function rule exists.
B. Yes. The data represents a functional relationship, but a function rule does not exist.
Incorrect. In a functional relationship, there is exactly one output value for every input value. Review the information in the table to determine if a function rule exists.
C. No. The data does not represent a function. However, there is a function rule. The function rule is y = 500x + 30000.
Incorrect. In a functional relationship, there is exactly one output value for every input value.
D. Yes. The data represents a functional relationship and there is a function rule. The function rule is y = 500x + 30000.
Correct! There is exactly one output value for every input value and a functional rule does exist.