In a proportional relationship, the two variables in the problem are related by a constant ratio. This means that the equation that relates the two variables can be written in the form:
y = constant • x, or y = k • x. 

When working with data in a table, the ratio must be the same for every pair of data points. It is important to calculate carefully so you can accurately compare the ratios.

One technique you can use is to reduce each ratio by factoring out the greatest common factor in both the numerator and the denominator. This will help you obtain a ratio reduced to lowest term.

Based on what you saw in the video, which pair of ratios are equivalent?
Interactive popup. Assistance may be required. Check Your Answer The ratio 20 25 is equivalent to the ratio 36 45 .

Why are they equivalent?
Interactive popup. Assistance may be required. Check Your Answer They both simplify to 4 5 .

Based on what you saw in the video, which pair of ratios are not equivalent?
Interactive popup. Assistance may be required. Check Your Answer The ratio 35 42 is not equivalent to the ratio 48 60 .

Why are they not equivalent?
Interactive popup. Assistance may be required. Check Your Answer They cannot be simplified to the same ratio.

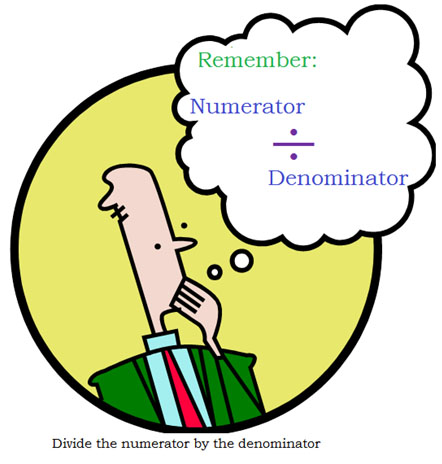
If the ratios are not easy to simplify, solve each one byInteractive popup. Assistance may be required. division and see if they are equal.

Read the following and decide if the ratios described by each pair of statements are equal or not equal.